WuTao's Blog

while(!(succeed = try()));

0%

2019 杭电多校 第一场

发表于 更新于 分类于 阅读次数： Valine：
本文字数： 5.6k 阅读时长 ≈ 5 分钟

2019 Multi-University Training Contest 1

补题链接：2019 Multi-University Training Contest 1

1002 Operation (HDU-6579)

题意

给定包含 $n$ 个数的序列，$m$ 个询问。询问有两种操作，操作 $0$ 表示在数组最后添加一个新元素，操作 $1$ 表示查询区间 [l,r] 的子集的异或最大值。

询问强制在线。

题解

线性基 贪心

1004 Vacation (HDU-6581)

题意

一条路上有 $n + 1$ 辆车。第 $i$ 辆车的长度为 $l_i$，离终点的距离为 $s_i$，最大车速为 $v_i$，$i$ 越大越靠近终点。每辆车不能超越前面的车，但车头可以贴在前面车的车尾。每辆车经过了终点，仍继续在路上跑。求第 $0$ 辆车 (最后一辆车) 车头通过终点线的最少需多少时间。

题解

贪心 二分

二分时间。判断 $mid = (l + r) / 2$ 时间能否最后一辆车到达，若能到达则 $r = mid$，否则 $l = mid$。

接下来是如何判断时间 $t$ 内最后一辆车能否到达终点。

从第一辆车 (最靠近终点) 开始枚举，维护一个 $cur$ 表示第 $i$ 辆车车头到终点的距离。第 $i$ 辆车经过 $t$ 时间后可以行驶 $car[i].v \times t$ 的距离，距离终点 $car[i].s - car[i].v \times t$。但是如果前面有车，就要比较前车车尾离终点的距离 $cur + car[i].l$ 谁更近。如果 $car[i].s - car[i].v \times t \le cur + car[i].l$，也就是第 $i$ 辆车行驶 $t$ 时间后距离终点更近，那么由于不能超过前车，也就是只能贴在前车的车尾，$cur$ 更新为前车车尾到终点的距离 $cur + car[i + 1].l$。如果 $car[i].s - car[i].v \times t > cur + car[i].l$，也就是不会超过前车，那么 $cur$ 就是当前车行驶 $t$ 时间后车头距离终点的距离。如果第 $0$ 辆车行驶 $t$ 时间后 $cur \le 0$，说明可以到达。

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
#include <bits/stdc++.h>
using namespace std;
const int maxn = 2e5 + 10;
const double eps = 1e-8;

struct CAR {
    int l, s, v;
} car[maxn];

int n;

int check(double x) {
    double cur = car[n].s - car[n].v * x;
    for (int i = n - 1; i >= 0; --i) {
        if (car[i].s - car[i].v * x <= cur + car[i + 1].l) cur += car[i + 1].l;
        else cur = car[i].s - car[i].v * x;
        if(cur > eps) return 0;
    }
    return cur <= eps;
}

int main() {
    while (~scanf("%d", &n)) {
        for(int i = 0; i < n + 1; ++i) {
            scanf("%d", &car[i].l);
        }
        for(int i = 0; i < n + 1; ++i) {
            scanf("%d", &car[i].s);
        }
        for(int i = 0; i < n + 1; ++i) {
            scanf("%d", &car[i].v);
        }
        double l = 0, r = 1e18;
        int cnt = 0;
        while (cnt < 100) {
            double mid = (l + r) / 2;
            if (check(mid)) r = mid;
            else l = mid;
            ++cnt;
        }
        printf("%.6lf\n", r);
    }
    return 0;
}

1005 Path (HDU-6582)

题意

给定 $n$ 个结点，$m$ 条边的有向图，现在要删除一些边，使得结点 $1$ 到 $n$ 的最短路的长度增加，删除边的代价为边的权值，求最少的代价。

题解

最短路 最小割

首先要找出所有的最短路，得到最短路图。对最短路图求最小割就是答案。

所有最短路的求法：先跑一遍单源最短路，可以用 $Dijkstra$ 算法。$Dijkstra$ 算法得到的是起点到所有点的最短距离，存放在 $d$ 数组中。那么遍历所有的边，如果 $d[i] + w_{ij} = d[j]$ ($w_{ij}$ 表示结点 $i$ 到结点 $j$ 的边的权值)，那么该条边一定在最短路图上。

至于最小割用 $Dinic$ 算法求解即可。

1013 Code (HDU-6590)

题意

给出两类点的坐标，问能否用一条直线将两类点分开。

题解

题目看懂了就很好做了。

就是分别对两类点求凸包，然后判断两个凸包是否相交。若不相交，则能够用一条直线分开两类点，否则不能。

其实就是判断凸包是否相交的模板题。

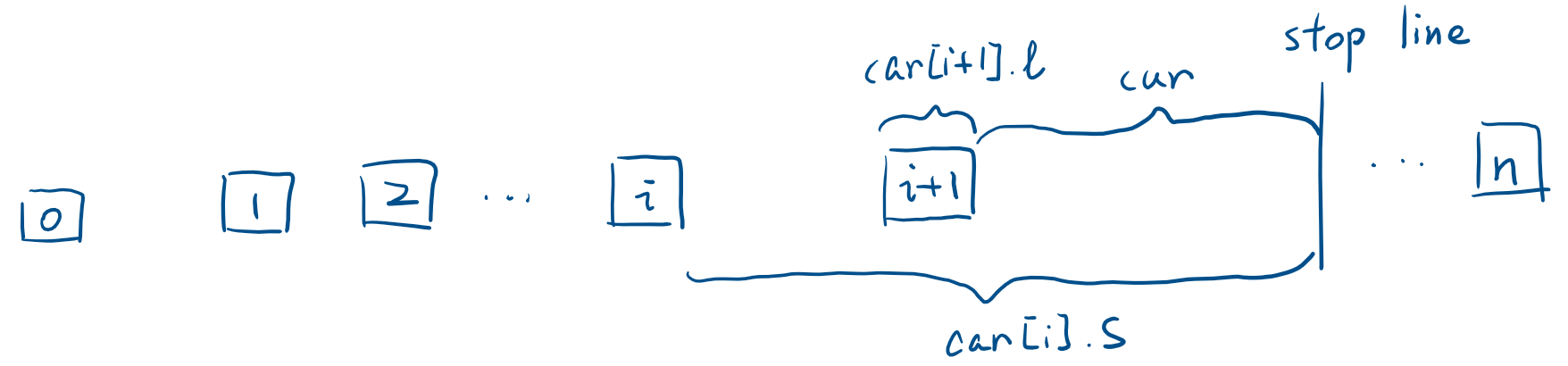
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
#include <bits/stdc++.h>
using namespace std;
const double eps = 1e-8;
const double pi = acos(-1.0);
class Point {
public:
    double x, y;
    Point(double x = 0, double y = 0) : x(x), y(y) {}
    Point operator+(Point a) {
        return Point(a.x + x, a.y + y);
    }
    Point operator-(Point a) {
        return Point(x - a.x, y - a.y);
    }
    bool operator<(const Point &a) const {
        if (x == a.x)
            return y < a.y;
        return x < a.x;
    }
    bool operator==(const Point &a) const {
        if (fabs(x - a.x) < eps && fabs(y - a.y) < eps)
            return 1;
        return 0;
    }
    double length() {
        return sqrt(x * x + y * y);
    }
};

typedef Point Vector;

double cross(Vector a, Vector b) {
    return a.x * b.y - a.y * b.x;
}

double dot(Vector a, Vector b) {
    return a.x * b.x + a.y * b.y;
}

bool isclock(Point p0, Point p1, Point p2) {
    Vector a = p1 - p0;
    Vector b = p2 - p0;
    if (cross(a, b) < -eps)
        return true;
    return false;
}

double getDistance(Point a, Point b) {
    return sqrt(pow(a.x - b.x, 2) + pow(a.y - b.y, 2));
}

typedef vector<Point> Polygon;
Polygon Andrew(Polygon s) {
    Polygon u, l;
    if(s.size() < 3) return s;
    sort(s.begin(), s.end());
    u.push_back(s[0]);
    u.push_back(s[1]);
    l.push_back(s[s.size() - 1]);
    l.push_back(s[s.size() - 2]);
    for(int i = 2 ; i < s.size() ; ++i) {
        for(int n = u.size() ; n >= 2 && !isclock(u[n - 2], u[n - 1], s[i]); --n) {
            u.pop_back();
        }
        u.push_back(s[i]);
    }
    for(int i = s.size() - 3 ; i >= 0 ; --i) {
        for(int n = l.size() ; n >=2 && !isclock(l[n-2],l[n-1],s[i]); --n) {
            l.pop_back();
        }
        l.push_back(s[i]);
    }
    for(int i = 1 ; i < u.size() - 1 ; i++) l.push_back(u[i]);
    return l;
}

int dcmp(double x)  {
    if (fabs(x) <= eps)
        return 0;
    return x > 0 ? 1 : -1;
}

// 判断点在线段上
bool OnSegment(Point p, Point a1, Point a2) {
    return dcmp(cross(a1 - p, a2 - p)) == 0 && dcmp(dot(a1 - p, a2 - p)) < 0;
}

// 判断线段相交
bool Intersection(Point a1, Point a2, Point b1, Point b2) {
    double c1 = cross(a2 - a1, b1 - a1), c2 = cross(a2 - a1, b2 - a1),
            c3 = cross(b2 - b1, a1 - b1), c4 = cross(b2 - b1, a2 - b1);
    return dcmp(c1) * dcmp(c2) < 0 && dcmp(c3) * dcmp(c4) < 0;
}

// 判断点在凸包内
int isPointInPolygon(Point p, vector<Point> s) {
    int wn = 0, cc = s.size();
    for (int i = 0; i < cc; i++) {
        Point p1 = s[i];
        Point p2 = s[(i + 1) % cc];
        if (p1 == p || p2 == p || OnSegment(p, p1, p2)) return -1;
        int k = dcmp(cross(p2 - p1, p - p1));
        int d1 = dcmp(p1.y - p.y);
        int d2 = dcmp(p2.y - p.y);
        if (k > 0 && d1 <= 0 && d2 > 0) wn++;
        if (k < 0 && d2 <= 0 && d1 > 0) wn--;
    }
    if (wn != 0) return 1;
    return 0;
}

void solve(Polygon s1, Polygon s2) {
    int c1 = s1.size(), c2 = s2.size();
    for(int i = 0; i < c1; ++i) {
        if(isPointInPolygon(s1[i], s2)) {
            printf("Infinite loop!\n");
            return;
        }
    }
    for(int i = 0; i < c2; ++i) {
        if(isPointInPolygon(s2[i], s1)) {
            printf("Infinite loop!\n");
            return;
        }
    }
    for (int i = 0; i < c1; i++) {
        for (int j = 0; j < c2; j++) {
            if (Intersection(s1[i], s1[(i + 1) % c1], s2[j], s2[(j + 1) % c2])) {
                printf("Infinite loop!\n");
                return;
            }
        }
    }
    printf("Successful!\n");
}

int main() {
    int T;
    cin >> T;
    while (T--) {
        int n;
        scanf("%d", &n);
        Polygon s1, s2;
        for (int i = 0; i < n; ++i) {
            double x1, x2, y;
            scanf("%lf%lf%lf", &x1, &x2, &y);
            if(y == 1) {
                s1.push_back(Point(x1, x2));
            } else {
                s2.push_back(Point(x1, x2));
            }
        }
        if(n == 1) {
            printf("Successful!\n");
            continue;
        }
        if(s1.size()) s1 = Andrew(s1);
        if(s2.size()) s2 = Andrew(s2);
        solve(s1, s2);
    }
    return 0;
}

欢迎关注我的其它发布渠道